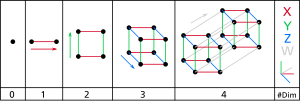In mathematics, a zero-dimensional topological space (or nildimensional space) is a topological space that has dimension zero with respect to one of several...

are one-dimensional spaces but are usually referred to by more specific terms. Any field K {\displaystyle K} is a one-dimensional vector space over itself...

case of metric spaces, (n + 1)-dimensional balls have n-dimensional boundaries, permitting an inductive definition based on the dimension of the boundaries...

A two-dimensional space is a mathematical space with two dimensions, meaning points have two degrees of freedom: their locations can be locally described...

0D (zero D) or 0-D may refer to: OD, IATA code for Darwin Airline Zero-day attack Zero-dimensional space 0x0D, the hex representation of newlines on some...

every zero-dimensional vector space. If all zero-dimensional vector spaces are assigned this orientation, then, because all isomorphisms among zero-dimensional...

0P (zero P) or 0-P may refer to: -polytope, a geometric form; see Zero-dimensional space 0-point energy or zero-point energy, the lowest possible energy...

three-dimensional Euclidean space, that is, the Euclidean space of dimension three, which models physical space. More general three-dimensional spaces are...

finite-dimensional if the dimension of V {\displaystyle V} is finite, and infinite-dimensional if its dimension is infinite. The dimension of the vector space V {\displaystyle...

Four-dimensional space (4D) is the mathematical extension of the concept of three-dimensional space (3D). Three-dimensional space is the simplest possible...