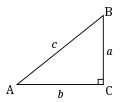
In geometry, a hypotenuse is the side of a right triangle opposite the right angle. It is the longest side of any such triangle; the two other shorter...

turn or 90 degrees). The side opposite to the right angle is called the hypotenuse (side c {\displaystyle c} in the figure). The sides adjacent to the right...

right triangle. It states that the area of the square whose side is the hypotenuse (the side opposite the right angle) is equal to the sum of the areas of...

side of the triangle (the hypotenuse), and the cosine is the ratio of the length of the adjacent leg to that of the hypotenuse. For an angle θ {\displaystyle...

a h {\displaystyle \sin \theta ={\frac {\mathrm {opposite} }{\mathrm {hypotenuse} }}={\frac {a}{h}}} cos ⁡ θ = a d j a c e n t h y p o t e n u s e = b...

the hypotenuse of the prior right triangle (with length the square root of 2) and the other leg having length of 1; the length of the hypotenuse of this...

vertex angle. In a right triangle, the altitude drawn to the hypotenuse c divides the hypotenuse into two segments of lengths p and q. If we denote the length...

the angle to the hypotenuse. sin ⁡ A = opposite hypotenuse = a h . {\displaystyle \sin A={\frac {\textrm {opposite}}{\textrm {hypotenuse}}}={\frac {a}{h}}...

the problem of finding equal products of a non-hypotenuse side and the hypotenuse, ( a 2 − b 2 ) ( a 2 + b 2 ) = ( c 2 − d 2 ) ( c 2 + d 2 )...

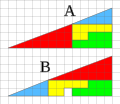
13x5 if it were, because what appears to be the hypotenuse is bent. In other words, the "hypotenuse" does not maintain a consistent slope, even though...