此條目介紹的是数学中的函数。关于程序设计中的函数,请见「
子程序 」。
函数 f {\displaystyle f} 黑箱 ,给予输入值 x {\displaystyle x} f ( x ) {\displaystyle f(x)} 函数 (英語:function )是數學 描述對應關係的一種特殊集合 ;粗略地說,從集合X Y Y X [ 2] X 定义域 [ 3] Y 到达域 。[ 4]
函數最初是一個變化的量如何依賴另一個量的理想化。例如,特定時間行星的位置可以視為是行星的位置對時間的函數。從歷史上看,這個概念是在17世紀末用無窮微積分來闡述的,直到19世紀,所考慮的函數都是可微的。函數的概念於19世紀末在集合論中被形式化,這大大擴展了這個概念的應用領域。
將形狀映射到其顏色的函數 若 x {\displaystyle x} 實數 ,以有序對 ( x , x 2 ) {\displaystyle (x,\,x^{2})} x {\displaystyle x} x 2 {\displaystyle x^{2}}
一般會以英文字母 f , g , h {\displaystyle f,\,g,\,h} x {\displaystyle x} f {\displaystyle f} f ( x ) {\displaystyle f(x)} f of x 」。函数的概念不限於數之间的對應关系,例定义函数 Capital {\displaystyle \operatorname {Capital} } 英国 就會输出唯一值伦敦 : Capital ( U . K . ) = L o n d o n {\displaystyle \operatorname {Capital} (\mathrm {U.K.} )=\mathrm {London} }
直觀上的「多變數函數」其實也可以概括到一般函數的定義裡。例如算式 x × y {\displaystyle x\times y} x {\displaystyle x} y {\displaystyle y} 有序对 ( x , y ) {\displaystyle (x,y)} ( ( x , y ) , x × y ) {\displaystyle ((x,\,y),\,x\times y)} f {\displaystyle f} f [ ( x , y ) ] = x × y {\displaystyle f[(x,\,y)]=x\times y} f ( x , y ) = x × y {\displaystyle f(x,\,y)=x\times y}
數學中,对应 、映射 、变换 通常都是函数的別稱,但也可能有別的意思,如在拓扑學 的映射有时代表的是连续函数 。
在類型論 的λ演算 中,「對應關係」可以是作為一個原始概念(也就是無定義名詞),而不像上述的定義把函數視為集合 的衍伸物。
函數的值域 或像 是定义域 中所有元素的像 之集合。[ 5] [ 6] [ 7] [ 8]
函数这个数学名词是莱布尼兹 在1694年开始使用的,用來描述跟曲线 相关的一個量,如曲线的斜率 或者曲线上的某一点。莱布尼兹所指的函数现在被称作可导函数 ,数学家之外的普通人一般接触到的函数即属此类。对于可导函数可以讨论它的极限 和导数 ,此两者描述了函数输出值的变化同输入值变化的关系,是微积分学 的基础。中文的「函数 」一词由清朝 数学家李善兰 译出。其《代数学》书中解释:「凡此變數中函(包含)彼變數者,則此為彼之函數」。
1718年,約翰·伯努利 把函数定义为「一个变量的函数是指由这个变量和常量以任何一种方式组成的一种量。」 1748年,伯努利的学生欧拉 在《无穷分析引论》一书中说:「一个变量 的函数是由该变量和一些数或常量 以任何一种方式构成的解析表达式 」,例如 f ( x ) = sin ( x ) + x 2 {\displaystyle f(x)=\sin(x)+x^{2}} 1775年,欧拉在《微分学原理》一书中又提出了函数的一个定义:「如果某些量以如下方式依赖于另一些量,即当后者变化时,前者本身也发生变化,则称前一些量是后一些量的函数。」 19世纪的数学家开始对数学的各个分支進行形式化。维尔斯特拉斯 倡議将微积分学建立在算术 ,而不是几何 的基础上,這種主張較趋向于欧拉的定义。 函数的定义得以擴展之後,数学家便能对一些「奇怪」的数学对象 进行研究,例如處處不可导的连续函数。这些函数曾经被认为只具有理论价值,迟至20世纪初时它们仍被视作「怪物」。稍后,人们发现这些函数在对如布朗运动 之类的物理现象进行建模时有重要的作用。 到19世纪末,数学家开始尝试利用集合论 来進行数学的形式化。他们试图将每一個数学对象都定义为集合 。狄利克雷 给出了现代正式的函数定义(参见下文#正式定義 )。在他的定義下,函数被视作数学关系 的特例。然而对于实际应用的情况,现代定义和欧拉定义的区别可以忽略不计。 函数f 的部分图像。每个实数的x 都与f (x ) = x 3 − 9x 相联系 定義 — 二元關係 f {\displaystyle f}
( ∀ x ) ( ∀ y ) ( ∀ y ′ ) { [ ( ⟨ x , y ⟩ ∈ f ) ∧ ( ⟨ x , y ′ ⟩ ∈ f ) ] ⇒ ( y = y ′ ) } {\displaystyle (\forall x)(\forall y)(\forall y^{\prime })\{\,[\,(\langle x,\,y\rangle \in f)\wedge (\langle x,\,y^{\prime }\rangle \in f)\,]\Rightarrow (y=y^{\prime })\,\}} 則稱為 f {\displaystyle f}
(為了避免 ( x , y ) {\displaystyle (x,\,y)} 逻辑 敘述的括弧混淆,也會用 ⟨ x , y ⟩ {\displaystyle \langle x,\,y\rangle } 有序对 )
也就是直觀上,有序对 ( x , y ) {\displaystyle (x,\,y)} 輸入值 , 輸出值 );而 f {\displaystyle f} 輸入值 , 輸出值 )來詳盡定義的對應規則,且每個輸入值只能對應一個輸出值 。
習慣上把 ( x , y ) ∈ f {\displaystyle (x,\,y)\in f} y = f ( x ) {\displaystyle y=f(x)} f ( x ) {\displaystyle f(x)} 一阶逻辑 公理化集合论 下額外新增的雙元函數符號 (因為 x {\displaystyle x} f {\displaystyle f}
公理 —
[ ¬ ( B ∧ C ) ∧ ( f ( x ) = ∅ ) ] ∨ [ ( B ∧ C ) ∧ ( ⟨ x , f ( x ) ⟩ ∈ f ) ] {\displaystyle [\,\neg ({\mathcal {B}}\wedge {\mathcal {C}})\wedge (f(x)=\varnothing )\,]\vee [\,({\mathcal {B}}\wedge {\mathcal {C}})\wedge (\langle x,\,f(x)\rangle \in f)\,]} 其中: B := ( ∀ x ) ( ∀ y ) ( ∀ y ′ ) { [ ( ⟨ x , y ⟩ ∈ f ) ∧ ( ⟨ x , y ′ ⟩ ∈ f ) ] ⇒ ( y = y ′ ) } {\displaystyle {\mathcal {B}}:=(\forall x)(\forall y)(\forall y^{\prime })\{\,[\,(\langle x,\,y\rangle \in f)\wedge (\langle x,\,y^{\prime }\rangle \in f)\,]\Rightarrow (y=y^{\prime })\,\}} f {\displaystyle f} C := ( ∃ y ) ( ⟨ x , y ⟩ ∈ f ) {\displaystyle {\mathcal {C}}:=(\exists y)(\langle x,\,y\rangle \in f)} x {\displaystyle x} f {\displaystyle f} 新增公理的合理性 假設有 B ∧ C {\displaystyle {\mathcal {B}}\wedge {\mathcal {C}}} 公式 B {\displaystyle {\mathcal {B}}} 量词公理A4 有: ( ∀ y ) ( ∀ y ′ ) { [ ( ⟨ x , y ⟩ ∈ f ) ∧ ( ⟨ x , y ′ ⟩ ∈ f ) ] ⇒ ( y = y ′ ) } {\displaystyle (\forall y)(\forall y^{\prime })\{\,[\,(\langle x,\,y\rangle \in f)\wedge (\langle x,\,y^{\prime }\rangle \in f)\,]\Rightarrow (y=y^{\prime })\,\}} 這樣綜合上式和 C {\displaystyle {\mathcal {C}}}
( ∃ ! y ) ( ⟨ x , y ⟩ ∈ f ) {\displaystyle (\exists !y)(\langle x,\,y\rangle \in f)} 換句話說:
B ∧ C ⊢ ( ∃ ! y ) ( ⟨ x , y ⟩ ∈ f ) {\displaystyle {\mathcal {B}}\wedge {\mathcal {C}}\vdash (\exists !y)(\langle x,\,y\rangle \in f)} 這樣根據特定條件下的存在性 就有:
⊢ ( ∃ ! y ) { [ ¬ ( B ∧ C ) ∧ ( y = ∅ ) ] ∨ [ ( B ∧ C ) ∧ ( ⟨ x , y ⟩ ∈ f ) ] } {\displaystyle \vdash (\exists !y)\{\,[\,\neg ({\mathcal {B}}\wedge {\mathcal {C}})\wedge (y=\varnothing )\,]\vee [\,({\mathcal {B}}\wedge {\mathcal {C}})\wedge (\langle x,\,y\rangle \in f)\,]\,\}} 這樣根據函數符號與唯一性 的內容,就可以於策梅洛-弗兰克尔集合论 增加上述的公理與雙元函數符號 f ( x ) {\displaystyle f(x)}
直觀上,這個公理表示「若 f {\displaystyle f} x {\displaystyle x} f {\displaystyle f} ⟨ x , f ( x ) ⟩ ∈ f {\displaystyle \langle x,\,f(x)\rangle \in f} f ( x ) {\displaystyle f(x)} 空集 」。
這樣根據函數符號與唯一性的定理(E) ,就會有本節一開始所說的直觀性質:
B ∧ C ⊢ ( ∀ y ) { [ y = f ( x ) ] ⇔ [ ⟨ x , y ⟩ ∈ f ] } {\displaystyle {\mathcal {B}}\land {\mathcal {C}}\vdash (\forall y)\{\,[\,y=f(x)\,]\Leftrightarrow [\,\langle x,\,y\rangle \in f\,]\,\}} 也就是「若 f {\displaystyle f} x {\displaystyle x} f {\displaystyle f} y {\displaystyle y} ⟨ x , y ⟩ ∈ f {\displaystyle \langle x,\,y\rangle \in f} y = f ( x ) {\displaystyle y=f(x)}
對於「n變數」的函數,也就是以
( ( x 1 , ⋯ , x n ) , y ) {\displaystyle ((x_{1},\,\cdots ,\,x_{n}),\,y)} 為元素的函數 f {\displaystyle {\mathcal {f}}} 項
f [ ( x 1 , ⋯ , x n ) ] {\displaystyle f[(x_{1},\cdots ,\,x_{n})]} 進一步簡寫為
f ( x 1 , ⋯ , x n ) {\displaystyle f(x_{1},\cdots ,\,x_{n})} 如果能指出函數 f {\displaystyle f} 公理化集合論 中,分类公理 確保對任意集合 A {\displaystyle A} D A {\displaystyle D_{A}} I A {\displaystyle I_{A}} 函數符號 )分別滿足
( ∀ x ) { ( x ∈ D A ) ⇔ ( ∃ y ) [ ( x , y ) ∈ A ] } {\displaystyle (\forall x)\{(x\in D_{A})\Leftrightarrow (\exists y)[\,(x,\,y)\in A\,]\}} ( ∀ y ) { ( y ∈ I A ) ⇔ ( ∃ x ) [ ( x , y ) ∈ A ] } {\displaystyle (\forall y)\{(y\in I_{A})\Leftrightarrow (\exists x)[\,(x,\,y)\in A\,]\}} 直觀上, D A {\displaystyle D_{A}} A {\displaystyle A} 有序对 的第一個所構成的集合; I A {\displaystyle I_{A}} A {\displaystyle A} 有序对 的第二個所構成的集合。這樣的話,如果 A {\displaystyle A} D A {\displaystyle D_{A}} 定義域 ;類似地, I A {\displaystyle I_{A}} 值域 。
通常情況下,有以下慣用的記號
f : X → Y := [ ( f is a function ) ∧ ( D f = X ) ∧ ( I f ⊆ Y ) ] {\displaystyle f:X\to Y:=[\,(f{\text{ is a function}})\wedge (D_{f}=X)\wedge (I_{f}\subseteq Y)\,]} 也就是直觀上, f : X → Y {\displaystyle f:X\to Y} f {\displaystyle f} 定義域 為 X {\displaystyle X} 值域 包含於 Y {\displaystyle Y} Y {\displaystyle Y} 對應域 。
屬於定義域 D f {\displaystyle D_{f}} x {\displaystyle x} 自變量 (independent variable),而項 f ( x ) {\displaystyle f(x)} 因變量( dependent variable),但是這跟實驗上的自变量和因变量 是稍有不同的,因為前者是現實得到的實驗值之間的關聯,但另一個是源於集合論的概念。
定義 — f {\displaystyle f}
( ∀ y ) ( ∀ x ) ( ∀ x ′ ) { [ ( ⟨ x , y ⟩ ∈ f ) ∧ ( ⟨ x ′ , y ⟩ ∈ f ) ] ⇒ ( x = x ′ ) } {\displaystyle (\forall y)(\forall x)(\forall x^{\prime })\{\,[\,(\langle x,\,y\rangle \in f)\wedge (\langle x^{\prime },\,y\rangle \in f)\,]\Rightarrow (x=x^{\prime })\,\}}
則被稱為一對一的 (one-to-one)或是单射 (injective function)。
直觀上,若函數 f {\displaystyle f} f {\displaystyle f}
若 f {\displaystyle f} 分类公理 所取的)以下的集合:
f − 1 := { p | ( ∃ x ) ( ∃ y ) [ p = ( y , x ) ∧ y = f ( x ) ] } {\displaystyle f^{-1}:=\{\,p\,|\,(\exists x)(\exists y)[\,p=(y,\,x)\,\wedge \,y=f(x)\,]\,\}} 也是一個函數,被稱為 f {\displaystyle f} 反函數 。
f : X → Y {\displaystyle f:X\to Y} Y {\displaystyle Y} 满射 (surjective function)定義為「 f : X → Y {\displaystyle f:X\to Y} 值域 就是 Y {\displaystyle Y}
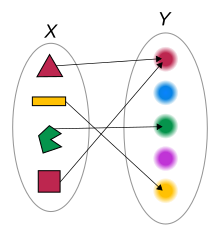
(1)一對多。X 中的元素3与Y 中的两个元素b 和c 相关。因此这是多值函数 ,而不是函数。
(2)一對一但非完全對應。X 的元素1未与Y 的任一元素相关。因此这是偏函数 ,而不是 函数。
(3)完全對應且多对一,因此这是从
X 到
Y 的函数。此函数可以表示为
f = { ( 1 , d ) , ( 2 , d ) , ( 3 , c ) } {\displaystyle f=\{\,(1,d),(2,d),(3,c)\}} ,或
f ( x ) = { d , if x = 1 d , if x = 2 c , if x = 3 {\displaystyle f(x)=\left\{{\begin{matrix}d,&{\mbox{if }}x=1\\d,&{\mbox{if }}x=2\\c,&{\mbox{if }}x=3\end{matrix}}\right.} 除了正式定義 一節所規範的集合論表示法,一般的數學書籍會採用比較通俗的函數表記方法,下面將一一介紹。
很多函數都是取实数 為輸出值和輸入值,換句話說,都是 f : A → R {\displaystyle f:A\to \mathbb {R} } A ⊆ R {\displaystyle A\subseteq \mathbb {R} } 实数加法 可由皮亚诺公理 裡的單元函數符號 S ( x ) {\displaystyle S(x)} x {\displaystyle x} x + 1 {\displaystyle x+1} 建構出來 ,或被視為实数公理系統 裡的雙元函數符號 P ( x , y ) {\displaystyle P(x,\,y)} x + y {\displaystyle x+y} 一阶逻辑 下的項 ;類似地,其他四則運算也可以此類推,而得出他們都是項 的結論。所以直觀上定義实数 函數的時候,都希望一條項(直觀上的運算式)能唯一決定一個函數,比如說,對於項 :
x + 1 {\displaystyle x+1} 以下的集合:
h := { p | ( ∃ x ∈ R ) [ p = ( x , x + 1 ) ] } {\displaystyle h:={\bigg \{}p\,{\bigg |}\,(\exists x\in \mathbb {R} )[p=(x,\,x+1)]{\bigg \}}} 是一個函數。為了讓這類函數的表示更加簡潔,就衍伸出以下的表記方式:
符號定義 — X 1 , … , X n {\displaystyle X_{1},\,\dots ,\,X_{n}} 集合 , T {\displaystyle T} x 1 , … , x n {\displaystyle x_{1},\,\dots ,\,x_{n}} 項 ,那在:
( ∀ x 1 ∈ X 1 ) … ( ∀ x n ∈ X n ) ( ∃ ! y ) ( y = T ) {\displaystyle (\forall x_{1}\in X_{1})\dots (\forall x_{n}\in X_{n})(\exists !y)(y=T)} 的前提下,則可做以下的符號定義:
f ( x 1 , … , x n ) = T ( ⟨ x 1 , … , x n ⟩ ∈ X 1 × ⋯ × X n ) {\displaystyle f(x_{1},\,\dots ,\,x_{n})=T\;\;(\langle x_{1},\,\dots ,\,x_{n}\rangle \in X_{1}\times \dots \times X_{n})\,} := {\displaystyle :=} f = { p | ∃ x 1 … ∃ x n [ ( ⟨ x 1 , … , x n ⟩ ∈ X 1 × ⋯ × X n ) ∧ ( p = ⟨ x 1 , … , x n , T ⟩ ) ] } {\displaystyle f={\bigg \{}p\,{\bigg |}\,\exists x_{1}\dots \exists x_{n}[(\langle x_{1},\,\dots ,\,x_{n}\rangle \in X_{1}\times \dots \times X_{n})\wedge (p=\langle x_{1},\,\dots ,\,x_{n},\,T\rangle )]{\bigg \}}} 這個表記方式被稱為函數記號 (functional notation),直觀上表示「若從 X i {\displaystyle X_{i}} x i {\displaystyle x_{i}} T {\displaystyle T} f ( x 1 , … , x n ) = T {\displaystyle f(x_{1},\,\dots ,\,x_{n})=T} T {\displaystyle T} T := x 1 + z {\displaystyle T:=x_{1}+z}
像是取 T {\displaystyle T} x + 1 {\displaystyle x+1}
( ∀ x ∈ R ) ( ∃ ! y ) ( y = x + 1 ) {\displaystyle (\forall x\in \mathbb {R} )(\exists !y)(y=x+1)} 因為單元對被規定成:
⟨ x ⟩ := x {\displaystyle \langle x\rangle :=x} 這樣就可以把前面的函數 h {\displaystyle h}
h ( x ) = x + 1 ( x ∈ R ) {\displaystyle h(x)=x+1\;(x\in \mathbb {R} )} 如果定義域可以從上下文推斷出來,函數記號可以更不正式的寫為:
f ( x 1 , … , x n ) = T {\displaystyle f(x_{1},\,\dots ,\,x_{n})=T} 比如說函數 h {\displaystyle h}
h ( x ) = x + 1 {\displaystyle h(x)=x+1} 這個記號是1734年第一次被萊昂哈德·歐拉 所採用[ 9] 項 與幂级数 ,因為當時並沒有一阶逻辑 這種清楚研究語言推理的系統;也並不知道有些物理 應用的函數不能用幂级数 展開[ 10]
以上的函數記號也可以稍作修改,來明確的指出「輸出值」的範圍:
符號定義 — X 1 , … , X n {\displaystyle X_{1},\,\dots ,\,X_{n}} Y {\displaystyle Y} 集合 , T {\displaystyle T} x 1 , … , x n {\displaystyle x_{1},\,\dots ,\,x_{n}} 項 ,在:
( ∀ x 1 ∈ X 1 ) … ( ∀ x n ∈ X n ) ( ∃ ! y ) [ ( y ∈ Y ) ∧ ( y = T ) ] {\displaystyle (\forall x_{1}\in X_{1})\dots (\forall x_{n}\in X_{n})(\exists !y)[\,(y\in Y)\wedge (y=T)\,]} 的前提下,可做以下的符號定義:
f : X 1 × ⋯ × X n → Y ; ⟨ x 1 , … , x n ⟩ ↦ T {\displaystyle f:X_{1}\times \dots \times X_{n}\to Y;\;\langle x_{1},\,\dots ,\,x_{n}\rangle \mapsto T} := {\displaystyle :=} f = { p | ∃ x 1 … ∃ x n [ ( ⟨ x 1 , … , x n ⟩ ∈ X 1 × ⋯ × X n ) ∧ ( p = ⟨ x 1 , … , x n , T ⟩ ) ∧ ( T ∈ Y ) ] } {\displaystyle f={\bigg \{}p\,{\bigg |}\,\exists x_{1}\dots \exists x_{n}[(\langle x_{1},\,\dots ,\,x_{n}\rangle \in X_{1}\times \dots \times X_{n})\wedge (p=\langle x_{1},\,\dots ,\,x_{n},\,T\rangle )\wedge (T\in Y)]{\bigg \}}} 這個表記方式被稱為箭號表示 (arrow notation),直觀上表示「若把從 X i {\displaystyle X_{i}} x i {\displaystyle x_{i}} T {\displaystyle T} Y {\displaystyle Y} X {\displaystyle X} Y {\displaystyle Y} ⟨ x 1 , … , x n ⟩ ↦ T {\displaystyle \langle x_{1},\,\dots ,\,x_{n}\rangle \mapsto T} f {\displaystyle f}
上述符號也可以比較通俗地記為:
f : X 1 × ⋯ × X n → Y ; f ( x 1 , … , x n ) = T {\displaystyle f:X_{1}\times \dots \times X_{n}\to Y;\;f(x_{1},\,\dots ,\,x_{n})=T} 比如說,取 T x {\displaystyle T_{x}} x + 1 {\displaystyle x+1}
( ∀ x ∈ R ) ( ∃ ! y ) [ ( y ∈ R ) ∧ ( y = x + 1 ) ] {\displaystyle (\forall x\in \mathbb {R} )(\exists !y)[(y\in \mathbb {R} )\wedge (y=x+1)]} 因為 x ∈ R {\displaystyle x\in \mathbb {R} } x + 1 ∈ R {\displaystyle x+1\in \mathbb {R} } h {\displaystyle h}
h : R → R ; h ( x ) = x + 1 {\displaystyle h:\mathbb {R} \to \mathbb {R} ;\;h(x)=x+1} 箭號表示常用來「固定」某個變數,來得到新的函數;假設 T x t {\displaystyle T_{xt}} x {\displaystyle x} t {\displaystyle t} 項 ,如果:
f : X × T → Y ; ( x , t ) ↦ T x t {\displaystyle f:X\times T\to Y;\;(x,t)\mapsto T_{xt}} τ ∈ T {\displaystyle \tau \in T} 那根據:
( ∀ x ) ( ∀ y ) ( ∀ x ′ ) ( ∀ y ′ ) { ( ⟨ x , y ⟩ = ⟨ x ′ , y ′ ⟩ ) ⇔ [ ( x = x ) ∧ ( y ′ = y ′ ) ] } {\displaystyle (\forall x)(\forall y)(\forall x^{\prime })(\forall y^{\prime })\{(\langle x,y\rangle =\langle x^{\prime },y^{\prime }\rangle )\Leftrightarrow [(x=x)\wedge (y^{\prime }=y^{\prime })]\}} 若假設 T x τ {\displaystyle T_{x\tau }} T x t {\displaystyle T_{xt}} t {\displaystyle t} τ {\displaystyle \tau } 項 ,那以下的符號簡寫也是可行的:
f τ : X → Y ; x ↦ T x τ {\displaystyle f_{\tau }:X\to Y;\;x\mapsto T_{x\tau }} 直觀上來說, f τ {\displaystyle f_{\tau }} f {\displaystyle f} t {\displaystyle t} τ {\displaystyle \tau } partial applied function 。
可以把箭號表示裡的 x {\displaystyle x} 间隔号 ,變成更通俗直觀的間隔號表示 ,比如說:
f : R → R ; x ↦ x 2 {\displaystyle f:\mathbb {R} \to \mathbb {R} ;\;x\mapsto x^{2}} 可以記為:
( ⋅ ) 2 {\displaystyle {(\cdot )}^{2}} 或是對於 [ a , b ] {\displaystyle [a,\,b]} 可積 的 f : [ a , b ] → R {\displaystyle f:[a,\,b]\to \mathbb {R} }
g : [ a , b ] → R ; x ↦ ∫ a x f ( u ) d u {\displaystyle g:[a,\,b]\to \mathbb {R} ;\;x\mapsto \int _{a}^{x}f(u)\,du} 函數 g {\displaystyle g}
∫ a ( ⋅ ) f ( u ) d u {\displaystyle \int _{a}^{(\cdot )}f(u)\,du} 但這個表記方法的明顯缺點是無法指出定義域,因為函數於哪個區間 可積 會決定以上的函數 g {\displaystyle g}
如果函數 f {\displaystyle f} f {\displaystyle f} 實函数 ),可以x軸代表定義域的範圍;y軸代表值域的範圍,把函數的每個元素標示在平面直角坐標 上,這被稱為實函数 f {\displaystyle f} 函數圖形 。
對於「雙變數」的實函數 g {\displaystyle g} x , y , z ∈ R {\displaystyle x,\,y,\,z\in \mathbb {R} }
( ( x , y ) , z ) {\displaystyle ((x,\,y),\,z)} 為元素的函數,可以取
D x = { x | ( ∃ y ) ( ∃ z ) [ g ( x , y ) = z ] } {\displaystyle D_{x}=\{\,x\,|\,(\exists y)(\exists z)[\,g(x,\,y)=z\,]\,\}} D y = { x | ( ∃ x ) ( ∃ z ) [ g ( x , y ) = z ] } {\displaystyle D_{y}=\{\,x\,|\,(\exists x)(\exists z)[\,g(x,\,y)=z\,]\,\}} 然後以x軸為 D x {\displaystyle D_{x}} D y {\displaystyle D_{y}} g {\displaystyle g} 直角坐標 繪出 g {\displaystyle g} 函數圖形 。
平面上的任意圖形可用豎直判别法 判斷是否為實函数 的圖形,即图形与任何一条平行于y轴的直线不能有一个以上的交點。但實際上這僅僅是函數正式定義的一種應用,因為平行于y轴的直线代表的是形如
{ p ∈ R 2 | ( ∃ y ∈ R ) [ p = ( c , y ) ] } {\displaystyle \{\,p\in {\mathbb {R} }^{2}\,|\,(\exists y\in \mathbb {R} )[\,p=(c,\,y)\,]\,\}} 的集合,也就是此直線交x軸於 ( c , 0 ) {\displaystyle (c,\,0)} f {\displaystyle f}
{ p ∈ R 2 | ( ∃ y ∈ R ) [ p = ( c , y ) ∧ y = f ( c ) ] } {\displaystyle \{\,p\in {\mathbb {R} }^{2}\,|\,(\exists y\in \mathbb {R} )[\,p=(c,\,y)\wedge y=f(c)\,]\,\}} 而屬於這個交集裡的平面點最多只能有一個 ,否則就會跟每個 x ∈ D f {\displaystyle x\in D_{f}} f ( x ) {\displaystyle f(x)}
像
第一種是形如 f ( x ) {\displaystyle f(x)} 項 ,直觀上代表的是依照函數 f {\displaystyle f} x {\displaystyle x} 函數值的簡記 )
第二種指的是集合 A {\displaystyle A} f {\displaystyle f} f ( A ) {\displaystyle f(A)}
f ( A ) := { y | ( ∃ x ∈ A ) [ y = f ( x ) ] } {\displaystyle f(A):=\{\,y\,|\,(\exists x\in A)[\,y=f(x)\,]\,\}} 注意 f {\displaystyle f} D f {\displaystyle D_{f}} f ( D f ) {\displaystyle f(D_{f})} 正式定義 一節的最後例子中, { 2 , 3 } {\displaystyle \{2,3\}} f {\displaystyle f} f ( { 2 , 3 } ) = { c , d } {\displaystyle f(\{2,3\})=\{c,d\}} f {\displaystyle f} { c , d } {\displaystyle \{c,d\}}
類似的,集合 B {\displaystyle B} f {\displaystyle f} 原像 (或逆像 )定義為:
f − 1 ( B ) := { x | ( ∃ y ) [ y = f ( x ) ∧ y ∈ B ] } {\displaystyle f^{-1}(B):=\{\,x\,|\,(\exists y)[\,y=f(x)\wedge y\in B\,]\,\}} 沿用同一例子,可以看到 { a , b } {\displaystyle \{a,b\}} f − 1 ( { a , b } ) = ∅ {\displaystyle f^{-1}(\{a,b\})=\varnothing } 空集 。
以下是 f {\displaystyle f} f − 1 {\displaystyle f^{-1}}
f ( A 1 ∪ A 2 ) = f ( A 1 ) ∪ f ( A 2 ) {\displaystyle f(A_{1}\cup A_{2})=f(A_{1})\cup f(A_{2})} f ( A 1 ∩ A 2 ) ⊆ f ( A 1 ) ∩ f ( A 2 ) {\displaystyle f(A_{1}\cap A_{2})\subseteq f(A_{1})\cap f(A_{2})} f ( B 1 ∪ B 2 ) = f − 1 ( B 1 ) ∪ f − 1 ( B 2 ) {\displaystyle f(B_{1}\cup B_{2})=f^{-1}(B_{1})\cup f^{-1}(B_{2})} f − 1 ( B 1 ∩ B 2 ) = f − 1 ( B 1 ) ∩ f − 1 ( B 2 ) {\displaystyle f^{-1}(B_{1}\cap B_{2})=f^{-1}(B_{1})\cap f^{-1}(B_{2})} f − 1 ( f ( B ) ) ⊆ B {\displaystyle f^{-1}(f(B))\subseteq B} f − 1 ( f ( A ) ) ⊇ A {\displaystyle f^{-1}(f(A))\supseteq A} 這些特性適合定義域的任意子集 A , A 1 {\displaystyle A,A_{1}} A 2 {\displaystyle A_{2}} 子集 B , B 1 {\displaystyle B,B_{1}} B 2 {\displaystyle B_{2}} 交集 和并集 。
若 f : X → Y {\displaystyle f:X\to Y} X ′ ⊂ X {\displaystyle X^{\prime }\subset X} f | X ′ {\displaystyle f|_{X^{\prime }}} × {\displaystyle \times } 笛卡儿积 )
f | X ′ := { ( x , y ) | ( x ∈ X ′ ) ∧ [ y = f ( x ) ] } = f ∩ ( X ′ × Y ) {\displaystyle f|_{X^{\prime }}:={\bigg \{}\,(x,\,y)\,{\bigg |}\,(x\in X^{\prime })\wedge [\,y=f(x)\,]\,{\bigg \}}=f\cap (X^{\prime }\times Y)} 顯然為一函數,稱為 f {\displaystyle f} X ′ {\displaystyle X^{\prime }} 限制
反之,若 g : X → Z {\displaystyle g:X\to Z} X ⊆ Y {\displaystyle X\subseteq Y} f : Y → Z {\displaystyle f:Y\to Z} f | X = g {\displaystyle f|_{X}=g} f {\displaystyle f} g {\displaystyle g} 擴張
設 f : X → R {\displaystyle f:X\to R} g : X → R {\displaystyle g:X\to R} ( R , + , × ) {\displaystyle (R,\,+,\,\times )} 環 。這樣可以定義「函數和」 f + g {\displaystyle f+g} f × g {\displaystyle f\times g}
f + g := { ( x , y ) | ( x ∈ X ) ∧ [ y = f ( x ) + g ( x ) ] } {\displaystyle {\begin{aligned}f+g:={\bigg \{}\,(x,\,y)\,{\bigg |}\,(x\in X)\wedge [\,y=f(x)+g(x)\,]\,{\bigg \}}\\\end{aligned}}} f + g := { ( x , y ) | ( x ∈ X ) ∧ [ y = f ( x ) × g ( x ) ] } {\displaystyle {\begin{aligned}f+g:={\bigg \{}\,(x,\,y)\,{\bigg |}\,(x\in X)\wedge [\,y=f(x)\times g(x)\,]\,{\bigg \}}\\\end{aligned}}} 很容易證明以上兩者也是函數,類似的對任意的 r ∈ R {\displaystyle r\in R}
r R := { ( x , y ) | ( x ∈ X ) ∧ ( y = r ) } {\displaystyle {\begin{aligned}r_{R}:={\bigg \{}\,(x,\,y)\,{\bigg |}\,(x\in X)\wedge (y=r)\,{\bigg \}}\\\end{aligned}}} r ⋅ f := { ( x , y ) | ( x ∈ X ) ∧ [ y = r × f ( x ) ] } {\displaystyle {\begin{aligned}r\cdot f:={\bigg \{}\,(x,\,y)\,{\bigg |}\,(x\in X)\wedge [\,y=r\times f(x)\,]\,{\bigg \}}\\\end{aligned}}} 也是函數,其中 r R {\displaystyle r_{R}} 常數函數 。
首都之於国家(若不把多首都国[ 註 1] 每个自然数 n {\displaystyle n} n 2 {\displaystyle n^{2}} n {\displaystyle n} 對數函數 。 ln x {\displaystyle \ln x} 正 实数 x {\displaystyle x} 對每个在 R 2 {\displaystyle \mathbb {R} ^{2}} 原点 ( 0 , 0 ) {\displaystyle (0,0)} 常用的数学函数包括多项式函數 、根式函數 、冪函數 、对数函數 、有理函数 、三角函数 、反三角函數 等。它们都是初等函数 。非初等函数(或特殊函数 )包括伽马函數 和贝塞尔函数 等。
函數可分為
在范畴论 中,函数的槪念被推廣為態射 的槪念。
一個范畴 包括一組物件與一組態射,每一個態射是個三元组(X , Y , f ),X 稱為源物件(定義域的類比),Y 稱為目標物件(到達域的類比),而源物件与目標物件是范畴內的物件。基于这种解释,可以把函数看作集合范畴 裡面的態射。
^ 1.0 1.1 Halmos, Paul R. Naive Set Theory . Springer-Verlag. 1970. ISBN 978-0-387-90092-6 ^ Halmos 1970 ,第30頁[ 1] map , mapping , transformation , correspondence , and operator are often used synonymously.^ Halmos 1970 [ 1] ^ Hazewinkel, Michiel (编), Mapping , 数学百科全书 , Springer , 2001, ISBN 978-1-55608-010-4 ^ Hazewinkel, Michiel (编), Function , 数学百科全书 , Springer , 2001, ISBN 978-1-55608-010-4 ^ Taalman, Laura; Kohn, Peter. Calculus . New York City : W. H. Freeman and Company . 2014. ISBN 978-1-4292-4186-1LCCN 2012947365 OCLC 856545590 OL 27544563M (英语) . ^ Trench, William F. Introduction to Real Analysis 2.04th. Pearson Education (originally; self-republished by the author). 2013 [2023-12-08 ] . ISBN 0-13-045786-8LCCN 2002032369 OCLC 953799815 Zbl 1204.00023 存档 于2023-11-23) (英语) . ^ Thomson, Brian S.; Bruckner, Judith B.; Bruckner, Andrew M. Elementary Real Analysis (PDF) 2nd. Prentice Hall (originally; 2nd ed. self-republished by the authors). 2008 [2023-12-08 ] . ISBN 978-1-4348-4367-8OCLC 1105855173 OL 31844948M Zbl 0872.26001 存档 (PDF) 于2023-05-26) (英语) . ^ Larson, Ron; Edwards, Bruce H. Calculus of a Single Variable. Cengage Learning. 2010: 19. ISBN 978-0-538-73552-0 ^ 曹, 亮吉. 數學導論. 科學月科社. 1988: 270–270, 277–277. Anton, Howard. Calculus with Analytical Geometry Wiley . 1980. ISBN 978-0-471-03248-9 Bartle, Robert G. The Elements of Real Analysis 2nd. Wiley. 1976. ISBN 978-0-471-05464-1 Dubinsky, Ed; Harel, Guershon. The Concept of Function: Aspects of Epistemology and Pedagogy. Mathematical Association of America. 1992. ISBN 978-0-88385-081-7 Hammack, Richard. 12. Functions (PDF) . Book of Proof . Virginia Commonwealth University . 2009 [2012-08-01 ] . (原始内容存档 于2024-06-08). Husch, Lawrence S. Visual Calculus . University of Tennessee . 2001 [2007-09-27 ] . (原始内容存档 于2011-09-24). Katz, Robert. Axiomatic Analysis. D. C. Heath and Company . 1964. Kleiner, Israel. Evolution of the Function Concept: A Brief Survey . The College Mathematics Journal. 1989, 20 (4): 282–300. CiteSeerX 10.1.1.113.6352 JSTOR 2686848 doi:10.2307/2686848 Lützen, Jesper. Between rigor and applications: Developments in the concept of function in mathematical analysis . Porter, Roy (编). The Cambridge History of Science: The modern physical and mathematical sciences. Cambridge University Press. 2003. ISBN 978-0-521-57199-9 Malik, M. A. Historical and pedagogical aspects of the definition of function. International Journal of Mathematical Education in Science and Technology. 1980, 11 (4): 489–492. doi:10.1080/0020739800110404 Reichenbach, Hans. Elements of Symbolic Logic. Dover. 1947. ISBN 0-486-24004-5 Ruthing, D. Old Intelligencer: Some definitions of the concept of function from Bernoulli, Joh. to Bourbaki, N. . Mathematical Intelligencer. 1984, 6 (4): 71–78. S2CID 189883712 doi:10.1007/BF03026743 Thomas, George B.; Finney, Ross L. Calculus and Analytic Geometry Addison-Wesley . 1995. ISBN 978-0-201-53174-9


 . Wiley. 1980. ISBN 978-0-471-03248-9.
. Wiley. 1980. ISBN 978-0-471-03248-9. . JSTOR 2686848. doi:10.2307/2686848.
. JSTOR 2686848. doi:10.2307/2686848. 9th. Addison-Wesley. 1995. ISBN 978-0-201-53174-9.
9th. Addison-Wesley. 1995. ISBN 978-0-201-53174-9.

 French
French Deutsch
Deutsch











![{\displaystyle f[(x,\,y)]=x\times y}](https://wikimedia.org/api/rest_v1/media/math/render/svg/051c520165328afe07bbb469d991726cd4008b55)


![{\displaystyle (\forall x)(\forall y)(\forall y^{\prime })\{\,[\,(\langle x,\,y\rangle \in f)\wedge (\langle x,\,y^{\prime }\rangle \in f)\,]\Rightarrow (y=y^{\prime })\,\}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/feb0e49c749d31216f0c6f103722cf8818d2d032)




![{\displaystyle [\,\neg ({\mathcal {B}}\wedge {\mathcal {C}})\wedge (f(x)=\varnothing )\,]\vee [\,({\mathcal {B}}\wedge {\mathcal {C}})\wedge (\langle x,\,f(x)\rangle \in f)\,]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/0218805d6da36b9d945595389f52acc561a9b959)
![{\displaystyle {\mathcal {B}}:=(\forall x)(\forall y)(\forall y^{\prime })\{\,[\,(\langle x,\,y\rangle \in f)\wedge (\langle x,\,y^{\prime }\rangle \in f)\,]\Rightarrow (y=y^{\prime })\,\}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/29f1bd046104550312151fd337ceff00efbd6b93)



![{\displaystyle (\forall y)(\forall y^{\prime })\{\,[\,(\langle x,\,y\rangle \in f)\wedge (\langle x,\,y^{\prime }\rangle \in f)\,]\Rightarrow (y=y^{\prime })\,\}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/f444a40dd1ed29cb8709db407edc64c69347e653)



![{\displaystyle \vdash (\exists !y)\{\,[\,\neg ({\mathcal {B}}\wedge {\mathcal {C}})\wedge (y=\varnothing )\,]\vee [\,({\mathcal {B}}\wedge {\mathcal {C}})\wedge (\langle x,\,y\rangle \in f)\,]\,\}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/7c16bf10a44f9ae2cadfd82648deb900a99f2502)

![{\displaystyle {\mathcal {B}}\land {\mathcal {C}}\vdash (\forall y)\{\,[\,y=f(x)\,]\Leftrightarrow [\,\langle x,\,y\rangle \in f\,]\,\}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/b1e62f49e2af8e48b0ce834bab48a443008b585e)



![{\displaystyle f[(x_{1},\cdots ,\,x_{n})]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/690eba035fa57cbdedc75bca64a84eaa4dd10b15)




![{\displaystyle (\forall x)\{(x\in D_{A})\Leftrightarrow (\exists y)[\,(x,\,y)\in A\,]\}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/be6b5a3bf44c87d590273c181ddd03e0137f7dae)
![{\displaystyle (\forall y)\{(y\in I_{A})\Leftrightarrow (\exists x)[\,(x,\,y)\in A\,]\}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/eacb3c9fa800bab536ed89e46e12652fe69917cb)
![{\displaystyle f:X\to Y:=[\,(f{\text{ is a function}})\wedge (D_{f}=X)\wedge (I_{f}\subseteq Y)\,]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/a5fd1fc7f2311a66db39ed44ebaf93cd06ecb117)




![{\displaystyle (\forall y)(\forall x)(\forall x^{\prime })\{\,[\,(\langle x,\,y\rangle \in f)\wedge (\langle x^{\prime },\,y\rangle \in f)\,]\Rightarrow (x=x^{\prime })\,\}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/c4be49f477df60724833a37416866a433b06a9d3)
![{\displaystyle f^{-1}:=\{\,p\,|\,(\exists x)(\exists y)[\,p=(y,\,x)\,\wedge \,y=f(x)\,]\,\}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/c98c482cef684eca46e2d6e58f4428914c1270c0)











![{\displaystyle h:={\bigg \{}p\,{\bigg |}\,(\exists x\in \mathbb {R} )[p=(x,\,x+1)]{\bigg \}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/b2f0f99901091b32d8109b762e046ff253e70747)






![{\displaystyle f={\bigg \{}p\,{\bigg |}\,\exists x_{1}\dots \exists x_{n}[(\langle x_{1},\,\dots ,\,x_{n}\rangle \in X_{1}\times \dots \times X_{n})\wedge (p=\langle x_{1},\,\dots ,\,x_{n},\,T\rangle )]{\bigg \}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/6ea396e7f24aaa2c086ad0d6fe35128a44ae8850)









![{\displaystyle (\forall x_{1}\in X_{1})\dots (\forall x_{n}\in X_{n})(\exists !y)[\,(y\in Y)\wedge (y=T)\,]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/dfcb7856433a72334794b10ccdce819328cf86b0)

![{\displaystyle f={\bigg \{}p\,{\bigg |}\,\exists x_{1}\dots \exists x_{n}[(\langle x_{1},\,\dots ,\,x_{n}\rangle \in X_{1}\times \dots \times X_{n})\wedge (p=\langle x_{1},\,\dots ,\,x_{n},\,T\rangle )\wedge (T\in Y)]{\bigg \}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/37edc0f7e0a1f90a100313ec347a2589679eb87c)



![{\displaystyle (\forall x\in \mathbb {R} )(\exists !y)[(y\in \mathbb {R} )\wedge (y=x+1)]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/2d8b1cad20d5537f2c8a5a9ad7c1ff42474c2884)







![{\displaystyle (\forall x)(\forall y)(\forall x^{\prime })(\forall y^{\prime })\{(\langle x,y\rangle =\langle x^{\prime },y^{\prime }\rangle )\Leftrightarrow [(x=x)\wedge (y^{\prime }=y^{\prime })]\}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/6aa1681c0724cae3298cf58872bd4c6338607817)






![{\displaystyle [a,\,b]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/b6403d6efced230f17c4b47aaf58879b629b048f)
![{\displaystyle f:[a,\,b]\to \mathbb {R} }](https://wikimedia.org/api/rest_v1/media/math/render/svg/ab904c8e15fe9ff81887ca14195f2712316da9c7)
![{\displaystyle g:[a,\,b]\to \mathbb {R} ;\;x\mapsto \int _{a}^{x}f(u)\,du}](https://wikimedia.org/api/rest_v1/media/math/render/svg/34de17cb8fd55bbbe4aceb146d99964811843199)




![{\displaystyle D_{x}=\{\,x\,|\,(\exists y)(\exists z)[\,g(x,\,y)=z\,]\,\}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/c9dddbd942ba946433d604d83c67676cfa21bd5c)
![{\displaystyle D_{y}=\{\,x\,|\,(\exists x)(\exists z)[\,g(x,\,y)=z\,]\,\}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/351b4bd689937b8a74fe68d440a6a71a62d98ce2)


![{\displaystyle \{\,p\in {\mathbb {R} }^{2}\,|\,(\exists y\in \mathbb {R} )[\,p=(c,\,y)\,]\,\}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/e3cab4f2ea350717f6ceecd749e509469bb84ce1)

![{\displaystyle \{\,p\in {\mathbb {R} }^{2}\,|\,(\exists y\in \mathbb {R} )[\,p=(c,\,y)\wedge y=f(c)\,]\,\}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/aa36ecb068d98412730298623c0693f4196404b6)


![{\displaystyle f(A):=\{\,y\,|\,(\exists x\in A)[\,y=f(x)\,]\,\}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/3e9d8b1aabbf585554b6bc4f1e1ac95a97d1a01b)





![{\displaystyle f^{-1}(B):=\{\,x\,|\,(\exists y)[\,y=f(x)\wedge y\in B\,]\,\}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/ac489453bbd5720b984a69f3bdd9b56cb17cfc46)
















![{\displaystyle f|_{X^{\prime }}:={\bigg \{}\,(x,\,y)\,{\bigg |}\,(x\in X^{\prime })\wedge [\,y=f(x)\,]\,{\bigg \}}=f\cap (X^{\prime }\times Y)}](https://wikimedia.org/api/rest_v1/media/math/render/svg/9326c2b80dccd3706cb754e74217e3318eafbc20)










![{\displaystyle {\begin{aligned}f+g:={\bigg \{}\,(x,\,y)\,{\bigg |}\,(x\in X)\wedge [\,y=f(x)+g(x)\,]\,{\bigg \}}\\\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/24cd44696743f359b814eaa20bcaaa58f683ea18)
![{\displaystyle {\begin{aligned}f+g:={\bigg \{}\,(x,\,y)\,{\bigg |}\,(x\in X)\wedge [\,y=f(x)\times g(x)\,]\,{\bigg \}}\\\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/e80221abfd7bf96f7b0c48b31cbe785b7158f908)


![{\displaystyle {\begin{aligned}r\cdot f:={\bigg \{}\,(x,\,y)\,{\bigg |}\,(x\in X)\wedge [\,y=r\times f(x)\,]\,{\bigg \}}\\\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/7d1a6a187fe46aab48c5b1d6460d8f54210ffb94)





