Tangens en cotangens

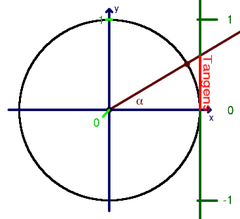
De tangens en cotangens zijn goniometrische functies, aangeduid met ${\displaystyle \tan }$ en ${\displaystyle \cot }$. Verouderde afkortingen ${\displaystyle \mathrm {tg} }$ en ${\displaystyle \mathrm {cotg} }$ komen nog wel voor. De naam tangens komt van raaklijn in het Latijn, tangens betekent rakend. Het argument van de tangens en de cotangens wordt vaak gezien als een hoek en dat heeft te maken met de oorspronkelijke definitie van deze functies. De tangens was gedefinieerd als de verhouding van de overstaande en de aanliggende rechthoekszijde in een rechthoekige driehoek. Deze oorspronkelijke definitie beperkte echter het domein van het argument van 0° tot 90°. Tot 90°, omdat de tangens voor 90° niet is gedefinieerd.

De inverse functie van de tangens is de arctangens of boogtangens, die voor een gegeven waarde van de tangens als functiewaarde de oorspronkelijke hoek, tussen −90° en +90°, geeft.

• 
  tangens van α in de eenheidscirkel
• 
  cotangens van α

De functiewaarde van de tangens loopt van ${\displaystyle 0}$ tot ${\displaystyle \infty }$ voor een argument lopend van 0° tot 90° en van ${\displaystyle -\infty }$ terug naar ${\displaystyle 0}$ voor een argument lopend van 90° tot 180°. Daarbuiten wordt de functie met een periode van 180° voortgezet. Ook is:

${\displaystyle \tan(-\alpha )=-\tan \alpha }$

Beide functies kunnen in de sinus en cosinus worden uitgedrukt:

${\displaystyle \tan \alpha ={\frac {\sin \alpha }{\cos \alpha }}}$

${\displaystyle \cot \alpha ={\frac {\cos \alpha }{\sin \alpha }}}$

De cotangens van een hoek is dus de omgekeerde van de tangens van die hoek, mits ${\displaystyle \cos \alpha \neq 0}$ en ${\displaystyle \sin \alpha \neq 0}$:

${\displaystyle \cot \alpha ={\frac {1}{\tan \alpha }}}$

graden	0°	30°	45°	60°	90°	120°	135°	150°	180°	270°
radialen	${\displaystyle 0\ }$	${\displaystyle {\tfrac {1}{6}}\pi }$	${\displaystyle {\tfrac {1}{4}}\pi }$	${\displaystyle {\tfrac {1}{3}}\pi }$	${\displaystyle {\tfrac {1}{2}}\pi }$	${\displaystyle {\tfrac {2}{3}}\pi }$	${\displaystyle {\tfrac {3}{4}}\pi }$	${\displaystyle {\tfrac {5}{6}}\pi }$	${\displaystyle \pi \ }$	${\displaystyle {\tfrac {3}{2}}\pi }$
tangens	${\displaystyle 0\ }$	${\displaystyle {\tfrac {1}{3}}{\sqrt {3}}}$	${\displaystyle 1\ }$	${\displaystyle {\sqrt {3}}}$	geen	${\displaystyle -{\sqrt {3}}}$	${\displaystyle -1\ }$	${\displaystyle -{\tfrac {1}{3}}{\sqrt {3}}}$	${\displaystyle 0\ }$	geen
cotangens	geen	${\displaystyle {\sqrt {3}}}$	${\displaystyle 1\,}$	${\displaystyle {\tfrac {1}{3}}{\sqrt {3}}}$	${\displaystyle 0\,}$	${\displaystyle -{\tfrac {1}{3}}{\sqrt {3}}}$	${\displaystyle -1\ }$	${\displaystyle -{\sqrt {3}}}$	geen	${\displaystyle 0\ }$

De tangens en cotangens kunnen ook in de vorm van een machtreeks geschreven worden, bijvoorbeeld als taylorreeks. Voor ${\displaystyle |x|<{\tfrac {\pi }{2}}}$ geldt:

${\displaystyle \tan x=x+{\tfrac {1}{3}}x^{3}+{\tfrac {2}{15}}x^{5}+{\tfrac {17}{315}}x^{7}+\ldots =\sum _{n=1}^{\infty }|B_{2n}|{\frac {2^{2n}\left(2^{2n}-1\right)}{(2n)!}}x^{2n-1}}$

${\displaystyle \cot x=x^{-1}-{\tfrac {1}{3}}x-{\tfrac {1}{45}}x^{3}-{\tfrac {2}{945}}x^{5}-\ldots =\sum _{n=0}^{\infty }(-1)^{n}B_{2n}{\frac {2^{2n}}{(2n)!}}x^{2n-1}}$

Daarin is ${\displaystyle B_{n}}$ het zogenaamde ${\displaystyle n}$-de bernoulligetal.

Beschouwt men ${\displaystyle x}$ als hoek, dan is ${\displaystyle x}$ uitgedrukt in radialen.

In een driehoek ${\displaystyle \triangle ABC}$ zonder rechte hoek geldt voor de grootte van de hoeken:

${\displaystyle \tan \alpha +\tan \beta +\tan \gamma =\tan \alpha \cdot \tan \beta \cdot \tan \gamma }$ en

${\displaystyle \cot {\frac {\alpha }{2}}+\cot {\frac {\beta }{2}}+\cot {\frac {\gamma }{2}}=\cot {\frac {\alpha }{2}}\cdot \cot {\frac {\beta }{2}}\cdot \cot {\frac {\gamma }{2}}}$

De tangensregel geldt voor alle driehoeken en geeft het volgende verband:

${\displaystyle {\frac {a-b}{a+b}}={\frac {\tan {\tfrac {1}{2}}(\alpha -\beta )}{\tan {\tfrac {1}{2}}(\alpha +\beta )}}}$

• [microcursus] goniometrie: sinus, cosinus, tangens (basis).
• Geometrie Tabel.