Archimedean solid - Simple English Wikipedia, the free encyclopedia

In geometry, an Archimedean solid is a convex shape which is composed of polygons. It is a polyhedron, with the following properties:

• Each face is made of a regular polygon
• All the corners of the shape look the same
• The shape is neither a platonic solid, nor a prism, nor an antiprism.

Depending on the way there are counted, there are thirteen or fifteen such shapes. Of two of these shapes, there are two versions, which cannot be made congruent using rotation. The Archimedean solids are named after the Ancient Greek mathematician Archimedes, who probably discovered them in the 3rd century BC. The writings of Archimedes have been lost, but Pappus of Alexandria summarized them in the 4th century.^[1] During the Renaissance, artists and mathematicians valued pure forms and rediscovered all of these forms. Johannes Kepler probably completed this search around 1620.^[2]

Constructing an Archimedean solid takes at least two different polygons.

• Archimedean solids are made of regular polygons, therefore all edges have the same length.
• All Archimedean solids can be produced from Platonic solids, by "cutting the edges" of the platonic solid.
• The type of polygons meting at a corner ("vertex") characterizes both the archimedean and platonic solid

Platonic solids can be turned into Archimedean solids by following a series of rules for their construction.

The following is a listing of all Archimedean solids

Image	Name	Faces	Type	Edges	Vertices
	Truncated tetrahedron	8	4 triangles 4 hexagons	18	12
	Cuboctahedron	14	8 triangles 6 squares	24	12
	Truncated cube	14	8 triangles 6 octagons	36	24
	Truncated octahedron	14	6 squares 8 hexagons	36	24
	Rhombicuboctahedron	26	8 triangles 18 squares	48	24
	Truncated cuboctahedron	26	12 squares 8 hexagons 6 octagons	72	48
	Snub cube (2 mirrored versions)	38	32 triangles 6 squares	60	24
	Icosidodecahedron	32	20 triangles 12 pentagons	60	30
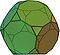	Truncated dodecahedron	32	20 triangles 12 decagons	90	60
	Truncated icosahedron	32	12 pentagons 20 hexagons	90	60
	Rhombicosidodecahedron	62	20 triangles 30 squares 12 pentagons	120	60
	Truncated icosidodecahedron	62	30 squares 20 hexagons 12 decagons	180	120
	Snub dodecahedron (2 mirrored versions)	92	80 triangles 12 pentagons	150	60